Question bank
Chapter-1 Patterns in Mathematics
Q-1
Find the pattern in the following sequences?
(a) 2, 2, 2, 2, 2, …………
(b) 10, 11, 12, 13, 14, …..
(c) 11, 13, 15, 17, ……
(d) 20, 22, 24, 26, 28, ……..
(e) 5, 10, 15, 20, 25, ……..
(f) 4, 16, 64, 256, 1024, ……
(g) 16, 25, 36, 49, ...
Q-2
Write the next three numbers for each of the following sequences.
(a) 11, 22, 33, 44, 55
(b) 1, 11, 21, 31, 41
(c) 1, 12, 123, 1234, 12345
(d) 5, 25, 125, 625, 3125
(e) 0, 1, 1, 2, 3, 5
Q-3
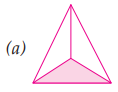
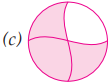
Draw the next picture for each of the following sequences:


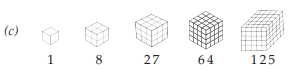

Q-4
Solve:
(a) Why are 1, 4, 9, 16, 25, … called square numbers or squares?
(b) Why are 1, 8, 27, 64, 125, … called cube numbers?
(c) Why are 1, 7, 19, 37, 61….. called hexagonal numbers?
Give two numbers greater than 36 which are triangular numbers as well as square numbers. [Hint: They are called hexagonal numbers.]
Q-5
Solve:
Q.1. Which sequence do you get when you start to add the counting numbers up? Give a pictorial explanation.
Q.2. What happens when you start to add up powers of 2 starting with 1. Now add 1 to each of these numbers — what numbers do you get? Explain it with a picture. Hint : 1 + 1 = 2; 1 + 2 + 1 = 4 = 2 x 2 etc.
Q.3. What happens when you multiply the triangular numbers by 6 and add 1? Which sequence do you get? Explain it with a picture? Hint : 1 × 6 + 1 = 7( increase by 6), 3 × 6 + 1 = 19 ( increase by 12) etc.
Q.4. Find your own patterns or relations in and among the sequences. Can you explain why they happen with a picture or otherwise?
Q-6
Solve:
Q.1. Count the number of lines in each shape in the sequence of complete graphs. Which number sequence do you get? Can you explain why?
Q.2. How many little squares are there in each shape of the sequence of Stacked Squares? Which number sequence does this give? Can you explain why?
Q.3. How many little triangles are there in each shape of the sequence of Stacked Triangles? Which number sequence does this give? Can you explain why? (Hint: In each shape in the sequence, how many triangles are there in each row?)
Multiple Choice Questions
Q-1 What is the pattern in the sequence 2, 2, 2, 2, 2, ....?
(i)
It increases by 1(ii)
It decreases by 1(iii)
All numbers are the same(iv)
The numbers alternateQ-2 What is the pattern in the sequence 10, 11, 12, 13, 14, ...?
(i)
It increases by 1(ii)
It increases by 2(iii)
It decreases by 1(iv)
It alternates between adding 1 and 2Q-3 What is the pattern in the sequence 11, 13, 15, 17, ......?
(i)
It increases by 1(ii)
It increases by 2(iii)
It increases by 3(iv)
It increases by 4Q-4 What is the pattern in the sequence 20, 22, 24, 26, 28, ......?
(i)
It increases by 1(ii)
It increases by 2(iii)
It increases by 3(iv)
It decreases by 2Q-5 What is the pattern in the sequence 5, 10, 15, 20, 25, ......?
(i)
It increases by 1(ii)
It increases by 2(iii)
It increases by 3(iv)
It increases by 5Q-6 What is the pattern in the sequence 4, 16, 64, 256, 1024, ......?
(i)
Each number is multiplied by 2(ii)
Each number is multiplied by 4(iii)
Each number is multiplied by 5(iv)
Each number is raised to the power of 4Q-7 What is the next number in the sequence 11, 22, 33, 44, 55?
(i)
60(ii)
66(iii)
77(iv)
65Q-8 Why are the numbers 1, 4, 9, 16, 25, ... called square numbers?
(i)
They are the result of multiplying a number by itself(ii)
They are the result of adding consecutive numbers(iii)
They are the result of multiplying two different numbers(iv)
They form a square shapeQ-9 What happens when you multiply triangular numbers by 6 and add 1?
(i)
You get a sequence of square numbers(ii)
You get a sequence of hexagonal numbers(iii)
You get a sequence of cube numbers(iv)
You get a sequence of prime numbersQ-10 How many little squares are there in each shape of the sequence of Stacked Squares?
(i)
The number increases by 1(ii)
The number increases by 2(iii)
The number increases by 3(iv)
The number increases by consecutive squaresChapter-2 Lines & Angles
Q-1
Classify the following angles as zero, acute, right, obtuse, straight, reflex and complete angles :
(a) 42°
(b) 92°
(c) 179°
(d) 205°
(e) 0°
(f) 79°
Q-2
Solve:
Q.1. Name the line given in all possible ways, choosing only two letters at a time from the four given.

Q.2. Answer the following questions : (a) What is the edge of brick like? (b) How many lines can be drawn through a point? (c) What is the tip of a cone like? (d) What are the points not located on the same line called?
Q.3. What is the difference between a ray and a line ? Draw a ray OB and a line segment OB.
Q.4. How many lines are drawn in the figure given below?
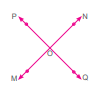
Q.5. Name this line in three different ways.

Q.6. In the adjoining figure, name (a) Four line segments (b) Four rays (c) Two non-intersecting line segments.
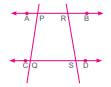
Q.7. Which of the following are models for perpendicular lines? (a) The adjacent edges of a blackboard (b) The opposite sides of a scale (c) The line segments forming the letter ‘H’ (d) The letter P (e) The adjacent edges of your book (f) The line segments forming the letter T (g) The line segments forming the letter Z
Q-3
Join A to other grid points in the figure by a straight line to get a
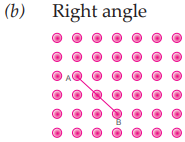

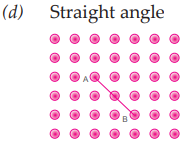

Q-4
Solve:
Q.1. Name the angles in the given figure
Q.2. Name three examples of angle from your daily life.
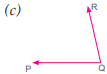
Q.3. In the given figure, name the points (a) In the interior of ∠XOZ. (b) In the exterior of ∠YOZ. (c) On ∠YOZ. (d) In the interior of ∠XOY.

Q.4. Write another name for the following angles in the given figure: (a) ∠1 (b) ∠2 (c) ∠3 (d) ∠4 (e) ∠5 (f) ∠6
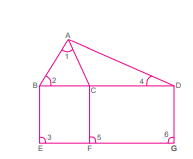
Q.5. In the given diagram, AC is a line. Are BA, BC and BD rays? There are three angles in the figure. One is ∠ABC. What are the others?
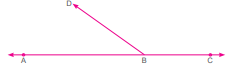
Q-5
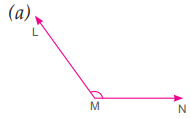
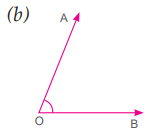
Using a protractor, measure the following angle.
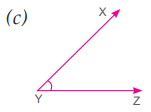
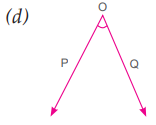
Q-6
Using a protractor, draw angles of the following measures:
(a) 30°
(b) 47°
(c) 90°
(d) 102°
(e) 140°
(f) 170°
Q-7
Solve:
Draw angles whose degree measure is the same as the angles given below.

Draw the letter ‘M’ such that the three angles formed are 60°, 120° and 60°.
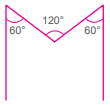
Q-8
By observation, classify the following angles as acute, right, obtuse, straight and reflex.

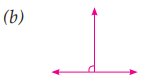


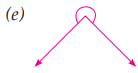
Q-9
Use a protractor to find the measure of each angle. Then classify each angle as acute, obtuse, right, or reflex.
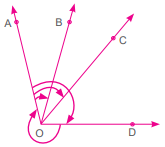
(a) ∠AOC
(b) ∠AOB
(c) ∠AOD
Q-10
Solve:
1. Find the degree measure of the angle given below using a protractor?
2. A wheel has 18 spokes. What is the degree measure of the angle between two spokes next to each other? What is the largest acute angle formed between two spokes?
3. Make any figure with two acute angles and three obtuse angles.
Multiple Choice Questions
Q-1 What is the edge of a brick like?
(i)
Curved(ii)
Straight(iii)
Pointed(iv)
CircularQ-2 How many lines can be drawn through a single point?
(i)
One(ii)
Two(iii)
Infinite(iv)
NoneQ-3 What is the tip of a cone like?
(i)
Flat(ii)
Pointed(iii)
Round(iv)
SquareQ-4 What are points that are not located on the same line called?
(i)
Collinear points(ii)
Non-collinear points(iii)
Intersecting points(iv)
Parallel pointsQ-5 Which of the following are models for perpendicular lines?
(i)
The adjacent edges of a blackboard(ii)
The line segments forming the letter H(iii)
The letter P(iv)
All of the aboveQ-6 Which of the following angles is classified as an obtuse angle?
(i)
30°(ii)
90°(iii)
120°(iv)
180°Q-7 What is the measure of the angle formed between two spokes of a wheel with 18 spokes?
(i)
10°(ii)
20°(iii)
30°(iv)
40°Q-8 Which of the following angles is a right angle?
(i)
45°(ii)
90°(iii)
120°(iv)
180°Q-9 If angle AOB measures 47°, how would you classify it?
(i)
Acute(ii)
Right(iii)
Obtuse(iv)
StraightQ-10 What is the measure of a complete angle?
(i)
90°(ii)
180°(iii)
360°(iv)
270°Chapter-3 Number Play
Q-1
Solve:
Q.1. Some children in a park are standing in a line. Each one speaks a number: 0 or 1 or 2 according to following code: ‘0’, if neither of the children standing next to them are taller. ‘1’ if there is only one taller child standing next to them. ‘2’ if both the children standing next to them are taller.
Fill in the speech bubbles by the number each child will speak.
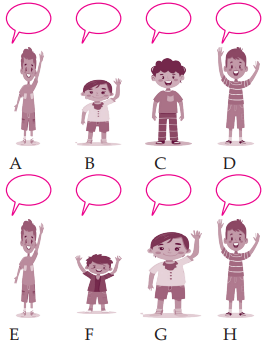
Q.2. Can the children rearrange themselves so that the children standing at the ends say ‘2’?
Q.3. Can we arrange the children in a line so that all would say only 0s?
Q.4. Can two children standing next to each other say the same number?
Q.5. Some children in a park are standing in a line. Each one speaks a number: 0 or 1 or 2 according to following code: ‘0’, if neither of the children standing next to them are shorter. ‘1’ if there is only one shorter child standing next to them. ‘2’ if both the children standing next to them are shorter.
Fill in the speech bubbles by the number each child will speak.
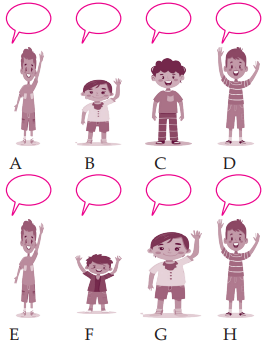
Q.6. There are 5 children in a group, all of different heights. Can they stand such that four of them say ‘1’ and the last one says ‘0’? Why or why not?
Q.7. For a group of six children, is the sequence 1, 1, 1, 1, 1, 1 possible?
Q.8. Is the sequence 0, 1, 2, 1, 0 possible? Why or why not?
Q.9. How would you rearrange the five children so that the maximum number of children say ‘2’?
Q-2
Solve the following questions:
Q.1. Colour the supercells in the table below.

Q.2. Colour the supercells in the table below.

Q.3. Colour the supercells in the table below.

Q.4. Check if the coloured cells are supercells.

Q.6. Write four digit numbers in blank boxes to make the coloured boxes as supercells.

Q.7. Fill the table below such that we get as many supercells as possible. Use numbers between 100 and 1000 without repetitions.

Q.10. Find out how many supercells are possible for different numbers of cells. Do you notice any pattern? What is the method to fill a given table to get the maximum number of supercells? Explore and share your strategy.
Q.11. Can you fill a supercell table without repeating numbers such that there are no supercells? Why or why not?
Q.12. Will the cell having the largest number in a table always be a supercell? Can the cell having the smallest number in a table be a supercell? Why or why not?
Q.13. Fill a table of 5 cells such that the cell having the second largest number is not a supercell.
Q.14. Fill a table of 5 cells such that the cell having the second smallest number is a supercell. Is it possible?
Q-3
Write the remaining numbers on the number lines given below. Put a circle around the smallest number and a box around the largest number in each of the sequences.



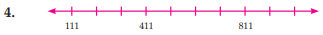
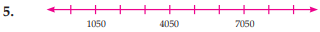
Q-4
Write two numbers whose sum of the digits is
(a) 11
(b) 12
Q-5
Write the smallest 4-digit number whose sum of the digit is (exclude 0)
(a) 13
(b) 14
Q-6
What is the largest 5-digit number whose digit sum is (exclude 0)
(a) 15
(b) 16
Q-7
Solve:
Find out the digit sums of all the numbers from 20 to 40.
Calculate the digit sums of all 3-digit numbers whose digits are consecutive (123, 234, …, 789). What pattern do you observe?
If we write all numbers from 1 to 100, how many times will 3 appear?
If we write all numbers from 1 to 100, how many times will 0 appear?
Q-8
Write all three-digit palindromes using
(a) 2, 3 and 4
(b) 5, 6 and 7
Q-9
Reverse and add the following till you get a palindrome:
(a) 23
(b) 37
(c) 57
Q-10
Check if by reversing and adding, you will get a palindrome:
(a) 231
(b) 162
(c) 465
Q-11
Staring with the given number and following the required steps reach 6174.
(a) 2397
(b) 9675
(c) 3456
Q-12
Solve:
1. How many rounds does the number 5683 take to reach the Kaprekar constant?
2. How many rounds does your year of birth take to reach the Kaprekar constant?
3. Find out the six times during the day the time written in 12 hour forms a palindromic number. (Hint: 11:11; 12:21 etc) How many times does it happen during a day?
4. Find out the six times during the day the time written in 24 hour forms a palindromic number. (Hint: 11:11; 12:21 etc) How many times does it happen during a day?
5. Write three dates written in dd-mm-yy format which form a palindrome?
6. Write three dates written in dd-mm-yyyy format which form a palindrome?
7. The time now is 10:01. How many minutes will the clock shows the next palindromic time? What about the one after that?
Q-13
Make the following numbers from the numbers in the middle? Use the figure given below.

(a) 3400
(b) 63,000
(c) 61,600
(d) 28,000
(e) 38,800
Q-14
Make the following numbers by adding and subtracting the given numbers.

(a) 45,000
(b) 5,900
(c) 17,500
(d) 21,400
Q-15
Write an example for each of the below scenarios whenever possible.
(a) 3-digit number + 3-digit number = 5-digit number
(b) 4-digit number + 4-digit number = 5-digit number
(c) 5-digit number + 5-digit number = 18,500
(d) 5-digit number – 5-digit number = 4-digit number
(e) 5-digit number + 5-digit number > 90,000
Q-16
Pratibha uses the digits ‘4’, ‘7’, ‘3’ and ‘2’, and makes the smallest and largest 4-digit numbers with them: 2347 and 7432. The difference between these two numbers is 7432 – 2347 = 5085. The sum of these two numbers is 9779. Choose 4–digits to make:
(a) the difference between the largest and smallest numbers greater than 5085.
(b) the difference between the largest and smallest numbers less than 5085.
(c) the sum of the largest and smallest numbers greater than 9779.
(d) the sum of the largest and smallest numbers less than 9779.
Q-17
Write one 5-digit number and two 4-digit numbers such that their sum is 18,670.
Q-18
Find the sum of numbers in the following pictures.
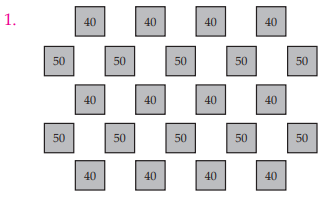
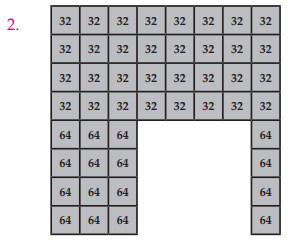
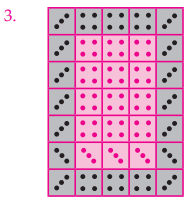
Q-19
Apply the Collatz conjecture on the following numbers and obtain the sequence.
(a) 17
(b) 21
(c) 22
(d) 34
Q-20
Solve:
1. Check if the Collatz Conjecture holds for the starting number 100.
2. Consider the sequence of powers of 2. Is the Collatz conjecture correct for all the starting numbers in this sequence? Why?
Q-21
Estimate the number of steps you would take to walk:
(a) across the school ground from start to end
(b) from the door of your home to a nearby shop.
(c) From your home to a nearby park.
Q-22
Number of times you blink your eyes:
(a) in a minute
(b) in an hour
(c) in a day
Q-23
Name some objects around you that are:
(a) hundreds in number
(b) thousands in number
(c) ten thousands in number
(d) lakhs in number
Q-24
Solve:
1. Estimate the number of words in your maths textbook: ( Hint: Count words on one page and multiply the number of pages)
2. Estimate the number of students in your school who travel to school by bus.
3. Estimate the distance between Chandigarh and Chennai. [Hint: Measure distance on a map. Use the scale provided in the map.] [Hint: Look at the map of India to locate these cities.]
4. Roshan wants to buy milk and 3 types of fruits to make fruit custard for 5 people. He estimates the cost to be ` 5000. Do you agree with him? Why or why not?
5. Sheetal is in Grade 6 and says she has studied for 15 hours in past one week. Do you agree with her? Why or why not?
6. Estimate the number of holidays you get in a year including weekends, festivals and vacation. Then try to get an exact number and see how close your estimate is.
7. Estimate the number of litres a mug, a bucket and an overhead tank can hold.
8. We are the group of 5-digit numbers between 35,000 and 75,000 such that all of our digits are odd. Who is the largest number in our group? Who is the smallest number in our group? Who among us is the closest to 50,000?
Q-25
Solve:
1. The first player says a number between 1 and 10. Then the two players take turns adding a number between 1 and 10 to the previous number said. The first player to reach 99 wins, Find a winning strategy for the above game?
2. Starting with 0, players alternate adding numbers between 1 and 3. The first person to reach 22 wins. What is the winning strategy now?
Multiple Choice Questions
Q-1 In a line of children, if one child says '1', how many of their neighbors are shorter?
(i)
None(ii)
One(iii)
Two(iv)
AllQ-2 What is the smallest 4-digit number?
(i)
1000(ii)
1001(iii)
2000(iv)
9999Q-3 What is the sum of the digits in the number 1234?
(i)
10(ii)
11(iii)
12(iv)
13Q-4 Which of the following is a palindromic number?
(i)
123(ii)
121(iii)
234(iv)
345Q-5 What is the result of adding two 3-digit numbers?
(i)
It can be a 3-digit number(ii)
It can be a 4-digit number(iii)
It can be both a 3-digit and a 4-digit number(iv)
It can never be a 3-digit numberQ-6 If the time is 10:01, what is the next palindromic time in 12-hour format?
(i)
10:10(ii)
11:11(iii)
12:21(iv)
10:11Q-7 What is the largest 5-digit number?
(i)
99999(ii)
100000(iii)
98765(iv)
99990Q-8 If a number is even, which of the following must be true?
(i)
It ends in 0, 2, 4, 6, or 8(ii)
It ends in 1, 3, 5, 7, or 9(iii)
It is a prime number(iv)
It is a multiple of 5Q-9 What is the sum of the angles in a quadrilateral?
(i)
180 degrees(ii)
360 degrees(iii)
90 degrees(iv)
270 degreesQ-10 Which of the following is a prime number?
(i)
15(ii)
21(iii)
29(iv)
35Q-11 If you reverse the digits of the number 321, what do you get?
(i)
123(ii)
321(iii)
213(iv)
132Q-12 What is the next number in the sequence: 2, 4, 6, 8, ...?
(i)
9(ii)
10(iii)
11(iv)
12Q-13 If a child is at the end of a line and says '0', what can we infer?
(i)
They are the tallest(ii)
They are the shortest(iii)
They are of equal height to their neighbor(iv)
They are shorter than their neighborQ-14 What is the digit sum of the number 456?
(i)
12(ii)
15(iii)
10(iv)
20Q-15 What is the next number in the sequence: 1, 3, 5, 7, ...?
(i)
8(ii)
9(iii)
10(iv)
11Chapter-4 Data Handling
Q-1
Define the terms:
(a) Data
(b) Raw data
(c) Array
Q-2
Following is the data collected from 50 students of their favourite fruits, a stands for mangoes, b for apples, c for oranges, d for bananas, e for grapes. Prepare a frequency chart.

Q-3
A dice was thrown 30 times and the following outcomes were noted: Represent the below data in the form of frequency distribution.

Q-4
The hourly wages of 30 workers (in rs) in a factory are given below. Make a table for the data using tally marks.

(a) What is the maximum daily wage of a worker?
(b) What is the minimum daily wage of a worker?
(c) How many workers are earning less than `50 per hour?
Q-5
Savi asked her students about the sizes of the shoes they wear. She noted the data on the board:
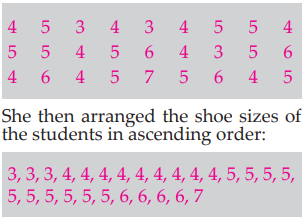
(a) Prepare a table for the above data.
(b) The largest shoe size in the class is _________
(c) The smallest shoe size in the class is _________
(d) There are _________ students who wear shoe size 5.
(e) There are ________ students who wear shoe sizes larger than 4.
(f) How did arranging the data in ascending order help to answer these questions?
Q-6
The numbers of fans produced by a factory in the four weeks of October are given below: Observe the pictograph and answer the following questions:

(a) In which week was the maximum number of fans produced? How many?
(b) In which week was the least number of fans produced? How many?
(c) How many fans were produced in the first week?
(d) What is the total number of fans produced in October?
Q-7
Following pictograph shows the number of tractors in five villages.
Observe the pictograph and answer the following questions:
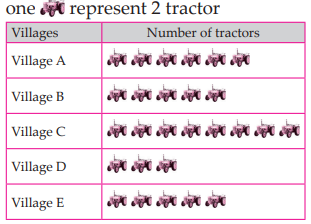
(a) Which village has the minimum number of tractors?
(b) Which village has the maximum number of tractors?
(c) How many more tractors village C has as compared to village B?
(d) What is the total number of tractors in all the five villages?
Q-8
The following pictograph shows the number of computers sold by a company during a week.
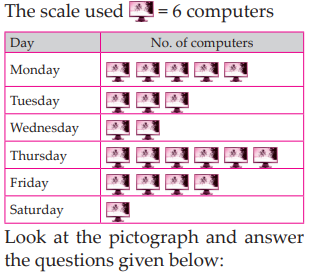
(a) How many computers were sold on Friday?
(b) How many computers were sold on Monday?
(c) How many computers were sold during the week?
(d) On which day was the maximum sale?
Q-9
The table below shows the number of students playing four different games.
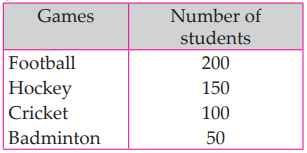
Present this information as a pictograph. You can use stickers to make the pictographs.
Q-10
In a monthly test, the numbers of students who passed in various subjects are given below:
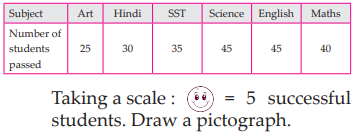
Q-11
Total numbers of animals in five villages are as follows:
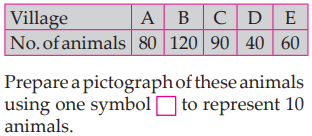
Q-12
The table below shows the number of cars sold in different cities in the month of August.
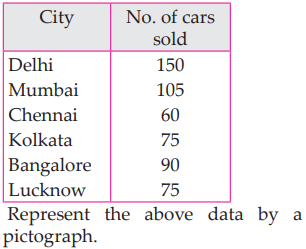
Q-13
The following graph represents the number of people of different countries in a world summit:
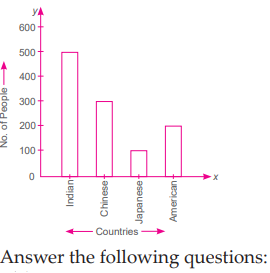
(a) How many Indians were present?
(b) Which country has minimum representatives?
(c) Which country has maximum representatives?
(d) Which countries have the same number of representatives?
(e) What is the total number of people in the summit?
Q-14
The bar graph given below shows the amount of wheat purchased in the years 1998-2002. Read the bar graph and write down your observations. In which year was
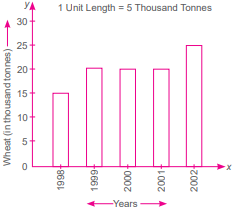
(a) The wheat purchased maximum?
(b) The wheat purchased minimum?
Q-15
In a survey of 160 families, the number of children in each family was recorded and the data has been represented by the bar graph, given below:
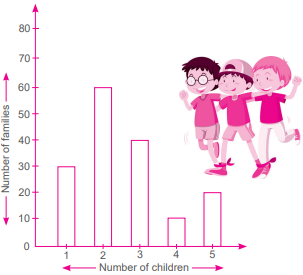
(a) What information does the bar graph give?
(b) How many families have 2 children, 3 children and 5 children?
(c) How many families have no child?
(d) What fraction of families have 4 children?
Q-16
51 students from a locality use different modes of transport to a school as shown by the bar graph given below:
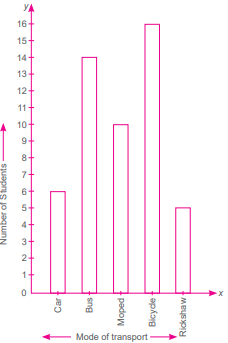
(a) What does the bar graph show?
(b) Which mode of transport is used by maximum number of students?
(c) How many students use bus for going to school?
(d) How many students of the locality do not use bus for going to school?
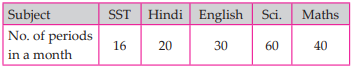
Q-17
Draw a bar graph to represent the data given below:
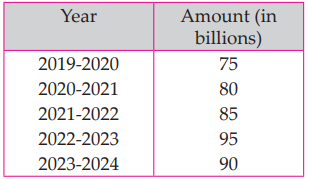
Q-18
India’s foreign trade export was as given below. Draw a bar graph to represent the data.
Q-19
The following table shows computer sets which are sold out during the week:
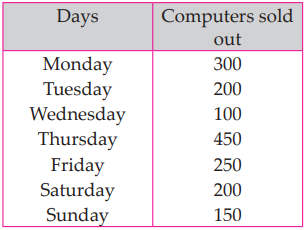
Draw a bar graph to represent the data.
Q-20
Solve:
1. Represent the following data on rivers and their lengths using infographics.

2. Represent the following data on metro cities of India and their population in millions, using infographics.

Multiple Choice Questions
Q-1 What is the term for a collection of numbers gathered to provide information?
(i)
Information(ii)
Data(iii)
Statistics(iv)
AnalysisQ-2 What do we call data that has not been processed or organized?
(i)
Processed data(ii)
Raw data(iii)
Structured data(iv)
Analyzed dataQ-3 Which of the following describes the arrangement of data in a systematic order?
(i)
Frequency(ii)
Array(iii)
Dataset(iv)
CollectionQ-4 What is the frequency of an observation?
(i)
The total number of observations(ii)
The average of the observations(iii)
The number of times a particular observation occurs(iv)
The highest value in the observationsQ-5 Which type of data is collected directly from the source?
(i)
Secondary data(ii)
Tertiary data(iii)
Primary data(iv)
Processed dataQ-6 What is the purpose of organizing data?
(i)
To make it more complex(ii)
To draw meaningful conclusions(iii)
To eliminate all errors(iv)
To increase the data sizeQ-7 Which of the following is a graphical representation of data?
(i)
Text document(ii)
Pictograph(iii)
Audio recording(iv)
SpreadsheetQ-8 What is the largest value in a dataset called?
(i)
Minimum(ii)
Mode(iii)
Maximum(iv)
MedianQ-9 What do we call the middle value in a sorted dataset?
(i)
Mean(ii)
Median(iii)
Mode(iv)
RangeQ-10 What is the term for the science that deals with the collection and analysis of numerical data?
(i)
Mathematics(ii)
Statistics(iii)
Data Science(iv)
EconomicsChapter-5 Prime Time
Q-1
Write all the factors of the following numbers :
(a) 21
(b) 24
(c) 27
(d) 36
(e) 60
(f) 64
Q-2
Write the first five multiples of:
(a) 5
(b) 6
(c) 7
(d) 8
(e) 12
Q-3
Match the items in column I with the items in column II.
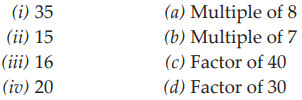
Q-4
Solve the following questions:
1. Find all the multiples of 8 between 60 and 100.
2. Find all multiples of 40 that lie between 270 and 410.
3. Find any three numbers that are multiples of 25 but not multiples of 50.
4. Find the smallest number that is a multiple of all the numbers from 1 to 10 except for 7.
Q-5
Solve these:
1. Write down separately the prime and composite numbers less than 20.
2. Check the following for being prime or composite : 23, 26, 31, 51, 109.
3. Write down the numbers between 10 and 30 which have exactly two factors.
4. Express each of the following numbers as the sum of two odd primes.36 and 98
5. Write seven consecutive numbers less than 100 so that there is no prime number between them.
Q-6
Express each of the following numbers as the sum of twin primes.
(a) 84
(b) 120
Q-7
Express each of the following numbers as the sum of three odd prime numbers.
(a) 49
(b) 35
Q-8
Solve the following questions:
Write three pairs of prime numbers less than 20 whose sum is a multiple of 5.
The numbers 13 and 31 are prime numbers. Both these numbers have same digits 1 and 3. Find such pairs of prime numbers up to 100.
Gita says, “Any two prime numbers are co-prime”. Is she correct?
Q-9
State True or False :
(a) All even numbers are composite. ____
(b) Odd numbers cannot be composite. ____
(c) The product of prime numbers cannot be prime. ____
(d) 1 is the smallest prime number. _____
(e) A number and its successor are always co-prime. _____
Q-10
Using divisibility test, determine which of the following numbers are divisible by 2.
(a) 5280
(b) 6427
(c) 7328
(d) 5193
Q-11
Using divisibility test, determine which of the following numbers are divisible by 4 and 8 :
(a) 572
(b) 726352
(c) 5500
(d) 1700
(e) 6000
Q-12
Using divisibility test, determine which of the following numbers are divisible by 6 :
(a) 297144
(b) 1258
(c) 4335
(d) 901350
(e) 2832
Q-13
Using divisibility test, determine which of the following numbers are divisible by 11:
(a) 5445
(b) 7138965
(c) 10824
(d) 70169308
Q-14
In each of the following, fill in the blanks with the smallest digit to make it divisible by 3 :
(a) _6724
(b) 75_3
(c) 135_8
(d) 206_38
Q-15
In each of the following, fill in the blanks with the smallest digit to make it divisible by 9 :
(a) 487_6
(b) 41_67
(c) 60_12
(d) 7_0514
Q-16
Solve:
1. By which digit should the box be replaced in 79 62 so that the resulting number is divisible by 11?
2. Find the largest and smallest 4-digit numbers that are divisible by 4 and are also palindromes
3. The teacher asked if 14560 is divisible by all of 2, 4, 5, 8 and 10. Guna checked for divisibility of 14560 by only two of these numbers and then declared that it was also divisible by all of them. What could those two numbers be?
Q-17
Express the following as a product of prime factors :
(a) 84
(b) 216
(c) 150
(d) 725
(e) 230
Q-18
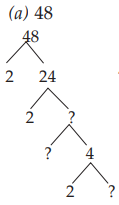
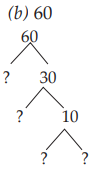
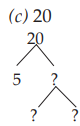
Fill up the factor tree given here and write down the prime factorisation for each.
Q-19
Solve:
1. Write the smallest 5-digit number and express it in the form of its prime factors.
2. Find the smallest number having four different prime factors.
Q-20
Find the common factors of :
(a) 12 and 15
(b) 10 and 28
(c) 15 and 25
(d) 56 and 120
(e) 8, 12, and 15
Q-21
Find first three common multiples of :
(a) 2 and 4
(b) 5 and 7
Q-22
Find the prime factorization of these numbers without multiplying.
(a) 56 × 25
(b) 108 × 75
(c) 1000 × 81
Q-23
Check if the first number divisible by the second? Use prime factorisation.
(a) 225 and 27
(b) 96 and 24
Multiple Choice Questions
Q-1 Which of the following numbers is a prime number?
(i)
15(ii)
21(iii)
23(iv)
25Q-2 What is the smallest prime number?
(i)
0(ii)
1(iii)
2(iv)
3Q-3 Which of the following statements is true?
(i)
All even numbers are prime.(ii)
All odd numbers are composite.(iii)
The product of two prime numbers is always prime.(iv)
1 is not a prime number.Q-4 What is the sum of the first two odd prime numbers?
(i)
4(ii)
5(iii)
10(iv)
6Q-5 Which of the following numbers is composite?
(i)
17(ii)
19(iii)
20(iv)
23Q-6 What is the greatest common factor (GCF) of 12 and 15?
(i)
1(ii)
3(iii)
4(iv)
6Q-7 Which of the following numbers is divisible by 4?
(i)
123(ii)
528(iii)
735(iv)
101Q-8 What is the first multiple of 7?
(i)
0(ii)
1(iii)
7(iv)
14Q-9 Which of the following is a factor of 36?
(i)
5(ii)
6(iii)
10(iv)
15Q-10 Which of the following numbers is divisible by 6?
(i)
123(ii)
234(iii)
345(iv)
456Chapter-6 Mensuration (Perimeter And Area)
Q-1
Measure and write the length of the four sides of a page of your notebook and find its perimeter.
P =___ cm +___ cm +___ cm +___ cm =___ cm
Q-2
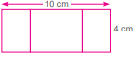
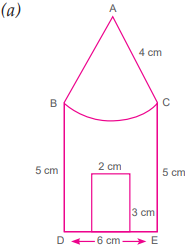
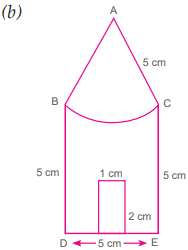
Find the perimeter of each of the following shapes:
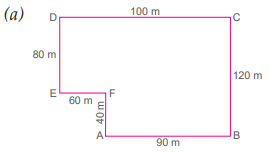
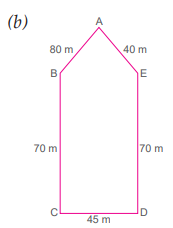
Q-3
Ritu went to a park 130 m long and 90 m wide. She took one complete round of it. What distance did she cover?

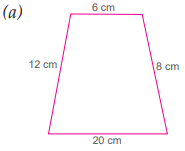
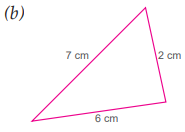
Q-4
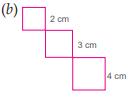
Find the perimeter of each of the following figures :
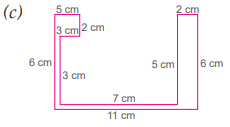
Q-5
Find the perimeter of a rectangle whose
(a)length = 34 m and breadth = 26 m
(b)length = 15.4 cm and breadth = 6.5 cm
Q-6
Solve the given questions:
1. Find the perimeter of a triangle with sides measuring 10 cm, 14 cm and 15 cm
2. Find the perimeter of a rectangular field which is 36 m long and 24 m wide.
3. Find the cost of fencing a rectangular field 24 m long and 18 m wide at `625 per metre.
4. An athlete takes 10 rounds of a rectangular park 50 m long and 25 m wide. Find the total distance covered by him.
5. A table top measures 3 m 75 cm by 2 m 70 cm. Find its perimeter.
6. Find the distance travelled by Shaina if she takes three rounds of a square park of side 70 m.
7. Tony runs around a square field of side 60 m, Shmeta runs around a rectangular field with length 80 m and breadth 50 m. Who runs the smaller distance?
8. Find the perimeter of a regular pentagon with each side measuring 3 cm.
9. Find the perimeter of a regular hexagon with each side measuring 8 m.
10. The perimeter of a rectangle is 1050 m and the breadth is 225 m. Find the length of the rectangle.
Q-7
Find the perimeter of a square whose side is
(a) 7 m
(b) 13 cm
(c) 1/56 cm
Q-8
A piece of wire is 24 cm long. What will be the length of each side, if the wire is used to form :
(a) an equilateral triangle
(b) a square
(c) a regular hexagon
Q-9
Find the perimeter of each of the following figures. How are the perimeters related with each other?
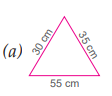
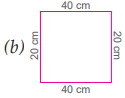
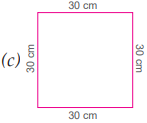
Q-10
Find the area of the rectangle with
(a) Length = 40 cm, Breadth = 20 cm
(b) Length = 20 m, Breadth = 30 m
(c) Length = 14.5 cm, Breadth = 8 cm
(d) Length = 80 m, Breadth = 75 m
Q-11
Find the area of the square with side
(a) 43 cm
(b) 5.6 cm
(c) 63 mm
(d) 2.6 cm
Q-12
Solve:
1. Find the area in square metre of a piece of cloth 1m 25 cm wide and 2m long.
2. The area of a rectangular garden 50 m long is 300 sq.m. Find the width of the garden
3. Five square flower beds each of sides 1 m are dug on a piece of land 5 m long and 4 m wide. What is the area of the remaining part of the land?
4. The cost of cementing the floor of a square room at the rate of `22 per sq.m is `550. Find the length of the room
5. How many square tiles with dimension 5 cm can be fitted in a small surface whose length is 50 cm and breadth is 25 cm?
6. The total cost of flooring a room at `850 per sq. metre is `51000. If the length of the room is 10 metres, find its breadth.
7. One metre is equal to 100 cm. How many sq. cm are there are in one sq.m?
8. How many tiles with dimensions 14 cm and 9 cm will be needed to fit a region whose length and breadth are 168 cm and 72 cm?
Multiple Choice Questions
Q-1 What is the formula for the perimeter of a rectangle?
(i)
Length + Breadth(ii)
2 — (Length + Breadth)(iii)
Length — Breadth(iv)
4 — SideQ-2 If the side of a square is 5 cm, what is its perimeter?
(i)
10 cm(ii)
15 cm(iii)
20 cm(iv)
25 cmQ-3 What is the area of a rectangle with a length of 10 m and a breadth of 5 m?
(i)
15 m²(ii)
50 m²(iii)
25 m²(iv)
30 m²Q-4 Which of the following shapes has a constant perimeter regardless of its size?
(i)
Rectangle(ii)
Square(iii)
Circle(iv)
TriangleQ-5 What is the area of a square with a side length of 4 m?
(i)
8 m²(ii)
12 m²(iii)
16 m²(iv)
20 m²Q-6 If a rectangular field is 30 m long and 20 m wide, what is its area?
(i)
600 m²(ii)
500 m²(iii)
400 m²(iv)
300 m²Q-7 What is the perimeter of a triangle with sides measuring 6 cm, 8 cm, and 10 cm?
(i)
24 cm(ii)
26 cm(iii)
28 cm(iv)
30 cmQ-8 How many square meters are there in 1 square kilometer?
(i)
1,000(ii)
10,000(iii)
100,000(iv)
1,000,000Q-9 If the length of a rectangular garden is doubled and the breadth remains the same, what happens to the area?
(i)
It remains the same(ii)
It doubles(iii)
It triples(iv)
It quadruplesQ-10 What is the area of a rectangle with a length of 12 cm and a breadth of 3 cm?
(i)
36 cm²(ii)
30 cm²(iii)
24 cm²(iv)
15 cm²Chapter-7 Fractions
Q-1
Which of the following are proper fractions?
(a) 15/8
(b) 1/2
(c) 7/4
(d) 6/19
Q-2
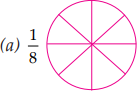
Write a fraction that represents the shaded part of each figure given below :

Q-3
Colour the part according to the given fraction.

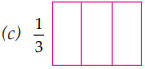
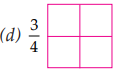
Q-4
Shade 1/3 portion of the figure.
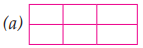

Q-5
Find numerator and denominator of each fraction :
(a) 4/10
(b) 6/7
(c) 8/5
(d) 3/13
Q-6
Write the fraction whose:
(a) N = 4, D = 6
(b) D = 3, N = 7
(c) N = 8, D = 5
(d) D = 7, N = 9
Q-7
Solve the following questions:
1. A wholesale merchant packed 1 kg of rice in 4 packets of equal weight. Find the weight of each packet in kg.
2. A family sized pizza was divided into 6 equal parts. What fraction of the pizza is each part?
3. Four friends ordered 3 glasses of sugarcane juice and shared it equally among themselves. What fraction of a glass of juice did each drink?
4. 4 m long piece of rope was cut into 5 equal parts. Find the length of each part in metres.
5. 3 kg of rice was divided in 4 equal parts. Find the weight of each part in kg
6. What fraction of a day is 8 hours?
7. What fraction of an hour is 40 minutes?
8. Write the natural numbers from 102 to 113. What fraction of them are prime numbers?
Q-8
Represent each of the following fractions on the number line :
(a) 1/3
(b) 1/4
(c) 5/7
(d) 4/9
Q-9
Insert >, = or < in the blank :
(a) 4/5___1
(b) 11/11___1
(c) 2/3____1
(d) 1___0/15
Q-10
Write the fraction that gives the lengths of the pink lines in the respective boxes.
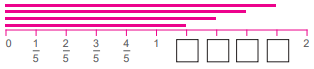
Q-11
Write fractions each with denominator 5 and less than 1.
Q-12
Fill in the blanks :
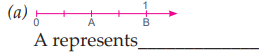

Q-13
Express the following as mixed fractions :
(a) 25/7
(b) 38/14
(c) 19/3
(d) 28/5
Q-14
Express the following as improper fractions :
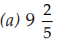
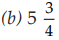
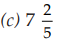
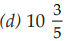
Q-15
Which of the following are improper fractions?
(a) 5/6
(b) 9/4
(c) 23/31
(d) 13/10
Q-16
Write five fractions equivalent to each of the following:
(a) 2/3
(b) 5/9
(c) 13/17
(d) 7/10
Q-17
Which of the following are the pairs of equivalent fractions?
(a) 5/6 and 20/24
(b) 4/7 and 16/21
(c) 4/5 and 16/25
Q-18
Find the equivalent fractions of 5/8 with
(a) Numerator 25
(b) Numerator 20
(c) Denominator 16
(d) Denominator 64
Q-19
Fill in the blanks :
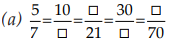
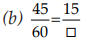
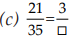
Q-20
Which of the following fractions are not in the simplest form? Reduce them to their simplest form.
(a) 15/14
(b) 25/56
(c) 20/84
(d) 63/34
Q-21
Solve:
Ramesh had 20 pencils, Sheela had 50 pencils and Jamaal had 80 pencils. After 4 months, Ramesh used up 10 pencils, Sheela used up 25 pencils and Jamaal used up 40 pencils. What fraction did each use up? Check if each has used up an equal fraction of her/his pencils?
Q-22
Match the equivalent fractions.
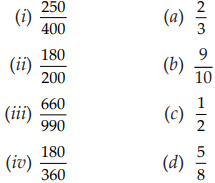
Q-23
Compare the fractions and put an appropriate sign (>, =, <) :
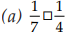
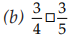
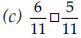
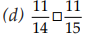
Q-24
In each of the groups identify like fractions :
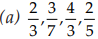
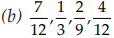
Q-25
Arrange the following in ascending order :
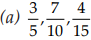
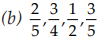
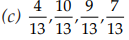
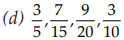
Q-26
Arrange the following in descending order:
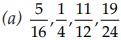
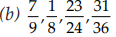
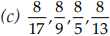
Q-27
Solve:
1. Rashmi read 25 pages of a book containing 100 pages. Pari read 2/5 of the same book. Who read less?
2. Luvnish exercised for 3/6 of an hour while Gagan exercised for 3/4 of an hour. Who exercised for a longer time?
3. Rachita walks 3/7 part of a certain distance and Mansi walks 5/8 part of the same distance in the same time. Who walks faster?
Q-28
Solve for the like fractions :
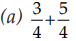
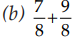
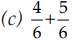
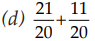
Q-29
Solve for the unlike fractions :
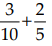
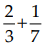
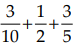
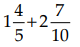
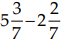
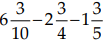
Q-30
Solve:
1. Renu did half of the work yesterday and one-third of the work today. How much work will she have to do tomorrow to complete the work?
2. In a vegetable garden 1/6 of the area is covered with lettuce and 5/12 with tomato plants. What fraction of the area is planted with these?
3. Of 5/7 and 9/14 , which is greater and by how much ?
Q-31
Solve:



Multiple Choice Questions
Q-1 What is the numerator in the fraction 3/5?
(i)
3(ii)
5(iii)
8(iv)
15Q-2 Which of the following is an example of a proper fraction?
(i)
15/8(ii)
1/2(iii)
7/4(iv)
6/19Q-3 What is the equivalent fraction of 1/2 with a numerator of 4?
(i)
2/4(ii)
4/8(iii)
4/2(iv)
8/4Q-4 Which of the following fractions is greater?
(i)
3/4(ii)
2/3(iii)
5/6(iv)
1/2Q-5 What is the sum of 1/4 and 1/4?
(i)
1/2(ii)
1/4(iii)
2/4(iv)
3/4Q-6 What fraction of an hour is 30 minutes?
(i)
1/2(ii)
1/3(iii)
1/4(iv)
2/3Q-7 Which of the following fractions is an improper fraction?
(i)
5/6(ii)
9/4(iii)
2/3(iv)
5/5Q-8 What is the mixed fraction of 11/4?
(i)
2 3/4(ii)
3 1/4(iii)
2 1/4(iv)
1 3/4Q-9 What is the fraction of a day that is 12 hours?
(i)
1/2(ii)
1/3(iii)
1/4(iv)
2/3Q-10 Which of the following fractions is equivalent to 2/3?
(i)
4/6(ii)
3/5(iii)
5/7(iv)
6/8Chapter-8 Constructions
Q-1
Using a scale only, draw a line segment of length:
(a) 5 cm
(b) 7 cm
(c) 9 cm
Q-2
Using ruler and compass, draw a line segment of length:
(a) 4 cm
(b) 6 cm
(c) 8 cm
Q-3
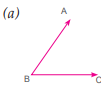
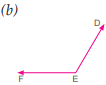
Measure the following angles using a protractor:
Q-4
Draw the angles of following measures using a protractor.
(a) 35°
(b) 145°
Q-5
Using a compass, draw a circle of radius:
a) 3 cm
b) 4 cm
c) 5 cm
Q-6
Solve:
Draw line ‘p’. Mark point P on it. Draw a perpendicular to ‘p’ at P.
Draw line ‘m’. Mark point M on it. Draw a perpendicular to ‘m’ at M.
Draw a line segment AB length 5 cm. Locate point X which is equidistant from A and B.
Q-7
Construct a rectangle with sides :
(a) 4 cm and 2 cm
(b) 5 cm and 3 cm
(c) 7 cm and 5 cm
Q-8
Construct a square of side:
(a) 3 cm
(b) 6 cm
(c) 7 cm
Q-9
Construct a rectangle that can be divided into two equal squares as shown in the figure.

Q-10
Construct a rectangle of sides 10 cm and 4 cm. Construct a square inside, as shown in the figure, such that the centre of the square is the same as the centre of the rectangle?
Q-11
Construct the following figure (Falling Squares):
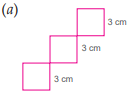
Q-12
solve:
1. Construct a square of side 5 cm. Make a circle in the centre of the square with radius 2 cm.
2. Construct a square of side 5 cm. Draw four arcs to bulge uniformly from each side
3. Construct a rectangle where one of its sides is 4 cm and the length of a diagonal is 6 cm.
Q-13
Construct a rectangle in which one of the diagonals divides the opposite angles into
(a) 60° and 30°
(b) 70° and 20°
Q-14
Draw the shape of an eye.
Q-15
Draw the following shapes of a person.
Q-16
Draw the following shapes of a house.
Multiple Choice Questions
Q-1 What is the primary purpose of using a compass in geometric constructions?
(i)
To measure angles(ii)
To draw straight lines(iii)
To draw circles and arcs(iv)
To measure lengthsQ-2 Which of the following describes a line segment?
(i)
A line that extends infinitely in both directions(ii)
A part of a line that has two endpoints(iii)
A line that curves(iv)
A point in spaceQ-3 What is the measure of a right angle?
(i)
30°(ii)
45°(iii)
90°(iv)
180°Q-4 If a rectangle has a length of 8 cm and a width of 4 cm, what is its perimeter?
(i)
24 cm(ii)
32 cm(iii)
20 cm(iv)
12 cmQ-5 What is the area of a square with a side length of 5 cm?
(i)
10 cm²(ii)
15 cm²(iii)
20 cm²(iv)
25 cm²Q-6 Which of the following statements is true about parallel lines?
(i)
They intersect at one point.(ii)
They are always the same length.(iii)
They never meet, no matter how far they are extended.(iv)
They form a right angle with each other.Q-7 What is the term for a figure that has four equal sides and four right angles?
(i)
Rectangle(ii)
Square(iii)
Rhombus(iv)
TrapezoidQ-8 If you need to construct a perpendicular line to a given line at a specific point, which geometric concept would you use?
(i)
Bisector(ii)
Angle(iii)
Intersection(iv)
Perpendicular bisectorQ-9 What is the relationship between the angles in a triangle?
(i)
The sum of the angles is 90°.(ii)
The sum of the angles is 180°.(iii)
The sum of the angles is 360°.(iv)
The angles are always equal.Q-10 Which of the following is NOT a type of angle?
(i)
Acute(ii)
Obtuse(iii)
Right(iv)
CircularChapter-9 Symmetry
Q-1
Draw the line of symmetry of each of the following figures :






Q-2
For each of the following letters, draw all lines of symmetry, if possible :





Q-3
Draw the other half of each of the figures to make it symmetrical:



Q-4
In each of the following figures, a hole was punched in a folded square sheet of paper and then the paper was unfolded. Identify the line along which the paper was folded. Figure (d) was created by punching a single hole. How was the paper folded?




Q-5
Can you draw a triangle which has
(a) Exactly one line of symmetry?
(b) Exactly two lines of symmetry?
(c) Exactly three lines of symmetry?
(d) No lines of symmetry?
Q-6
How many lines of symmetry can be drawn through:
(a) an oval
(b) the English letter C
(c) an isosceles trapezium
(d) a regular pentagon
(e) a square
Q-7
Solve:
1. Identify any three letters of the English alphabet through which no line of symmetry can be drawn.
2. Mark two points X and Y 6.5 cm apart. Draw a line AB so that the two points marked as X and Y are symmetric with respect to the line AB. Give a special name to line AB.
3. Write all letters of English alphabet which have more than one lines of symmetry.
Q-8
Study the letters given below:

(a) Which two letters have more than one line of symmetry?
(b) Which letter has no line of symmetry?
Q-9
Draw the following. In each case, the figure should contain at least one curved boundary.
(a) A figure with exactly one line of symmetry.
(b) A figure with exactly two lines of symmetry.
(c) A figure with exactly four lines of symmetry.
Q-10
Solve the following question:
1. What is the order of rotational symmetry of a square? Give the angles of such rotations.
2. Write three letters of the English alphabet which have two lines of symmetry and rotational symmetry of order 2.
3. If after rotating by 40o about its centre a figure looks exactly the same as at its original position, at what other angle will this happen again for the figure?
4. In a figure, 60° is an angle of symmetry. The figure has two angles of symmetry less than 60°. What is its smallest angle of symmetry?
Q-11
Draw, wherever possible, a rough sketch of
(a) A triangle with at least two lines of symmetry and at least two angles of symmetry.
(b) A triangle with only one line of symmetry but not having rotational symmetry.
(c) A quadrilateral with rotational symmetry but no reflection symmetry.
(d) A quadrilateral with reflection symmetry but not having rotational symmetry of order more than 1.
Q-12
Solve:
1. If a figure has two or more lines of symmetry, should it have rotational symmetry of order more than 1 ?
2. Does every trapezium have a line of symmetry?
3. Give an example of a geometrical figure which has no a line of symmetry but rotational symmetry of order 1.
4. A circular wheel has eight spikes. What is its angle of rotation and the order of rotational symmetry?
Q-13
Fill in the blanks.
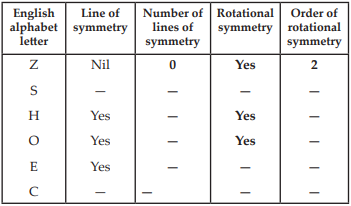
Multiple Choice Questions
Q-1 What is symmetry?
(i)
A measure of size(ii)
A balanced arrangement of parts(iii)
A type of angle(iv)
A method of drawingQ-2 Which of the following letters has vertical line symmetry?
(i)
B(ii)
C(iii)
A(iv)
DQ-3 What is the line that divides a figure into two equal halves called?
(i)
Line of reflection(ii)
Line of symmetry(iii)
Line of proportion(iv)
Line of balanceQ-4 Which of the following shapes has no line of symmetry?
(i)
Circle(ii)
Square(iii)
Triangle(iv)
Scalene triangleQ-5 How many lines of symmetry does a rectangle have?
(i)
1(ii)
2(iii)
4(iv)
0Q-6 Which of the following letters has horizontal line symmetry?
(i)
M(ii)
U(iii)
H(iv)
KQ-7 If a figure can be folded along a line and both halves match exactly, it is said to be:
(i)
Asymmetrical(ii)
Symmetrical(iii)
Irregular(iv)
CongruentQ-8 Which of the following shapes has infinite lines of symmetry?
(i)
Square(ii)
Circle(iii)
Triangle(iv)
RectangleQ-9 Which of the following letters does NOT have any line of symmetry?
(i)
O(ii)
X(iii)
Z(iv)
TQ-10 What type of symmetry does the letter 'M' exhibit?
(i)
Horizontal symmetry(ii)
Vertical symmetry(iii)
Rotational symmetry(iv)
No symmetryChapter-10 Integers
Q-1
Use the number line to write points corresponding to each of the following :

(a) Opposite of 7
(b) 3 units less than 4
(c) 6 units less than 1
(d) Opposite of A
Q-2
Insert > or < sign in the box :
(a) – 6 ___ – 8
(b) – 2 ___ – 1
(c) – 15 ___ – 7
(d) – 11 ___ – 10
Q-3
Arrange the following numbers in ascending order :
(a) – 4, – 125, – 21, – 60, – 48
(b) – 98, – 75, – 14, – 32, – 47
Q-4
Give the opposites of :
(a) Increase in population
(b) 300 BCE
Q-5
Arrange the following numbers in descending order :
(a) – 2, – 15, – 53, – 26, – 85
(b) – 27, – 76, – 115, – 36, – 160
Q-6
Represent the following numbers on a number line :
(a) + 5
(b) – 10
(c) + 8
(d) – 1
Q-7
Identify the larger integer in each of the following pairs of integers :
(a) 1, 6
(b) – 1, – 6
(c) 0, 4
(d) 5, 7
Q-8
Using the number line write >, = or < in the boxes in each case :
(a) 0 ___ – 3
(b) – 4 ___ 1
(c) – 7 ___ – 5
(d) – 10 ___ – 10
Q-9
Solve:
1. Write four negative integers greater than – 20.
2. Write four negative integers less than – 10.
3. How many integers are between –3 and 5? Write them in order.
Q-10
Write the following in ascending order :
(a) |– 18|, |– 64|, |– 13|, |– 46|, |– 72|
(b) |48|, |– 47|, – |– 54|, – | – 30 |, |– 72|
Q-11
Write the following in descending order :
(a) |– 32|, | – 68 |, |–75|, |80|, –|52|
(b) |48|, – 47, – |– 52|, – |– 28|, |– 50|
Q-12
Find the value of:
(a) |– 49|
(b) |– 3546|
(c) |9 – 8|
(d) 25 – |– 12| + |– 10|
Q-13
Write absolute value of each :
(a) 6
(b) – 7
(c) 0
(d) – 193
Q-14
Add the following integers :
(a) – 410 and 172
(b) 120 and – 10
(c) – 88 and – 43
(d) 200 and – 75
Q-15
Subtract the following integers :
(a) + 11 from + 5
(b) – 6 from – 14
(c) + 114 from + 1024
(d) + 68 from – 32
Q-16
Simplify.
(a) (– 4) + 12 + (– 20)
(b) 41 + (– 11) + (– 17)
(c) (– 200) + 110 + (– 20) + 210 + (– 5)
(d) (– 7) + 5 + (– 8) + (– 11)
Q-17
Solve the following question:
1. Subtract 211 from the difference of –20 and –57.
2. Add – 41 to the difference of –220 and – 176.
3. The sum of two integers is – 20. If one of the integers is 120, find the other.
4. The sum of two integers is 88. If one of the integers is – 28, find the other.
5. Suraj travelled 32 km north and Ashish travelled 74 km south from the same point. Find the distance between the destinations of the two.
6. Critical Thinking : What is the difference between the successor and the predecessor of an integer ? Can we generalise it as a rule ?
7. At 5 p.m., the temperature was 22°C above zero. At 2 a.m., the temperature was 1°C below zero. Find the change in the temperature.
8. The point A is 27,500 metres below sea level and the point B, on a mountain, is 2,000 metres above sea level, find the vertical distance between A and B.
9. Temperature of a place at noon was +5°C. Temperature increased by 3°C in first hour and decreased by 1°C in the second hour. What was the temperature at 2:00 pm?
10. An aircraft was flying at a height of 4,000 feet. The pilot decreased the height by 500 feet. Then he increased the height by 700 feet. At what height is the plane flying now?
11. Find two integers with different signs whose sum is – 81.
12. Find two integers with different signs whose difference is – 25.
13. In Antarctica desert, the temperatures near the coast reach 12°C in the summer and – 45°C in the winters. Find the range of the temperature.
Multiple Choice Questions
Q-1 Which of the following is an integer?
(i)
3.5(ii)
-2(iii)
0.75(iv)
5.1Q-2 What is the absolute value of -15?
(i)
-15(ii)
0(iii)
15(iv)
-1Q-3 Which of the following pairs of numbers are opposites?
(i)
3 and 3(ii)
-5 and 5(iii)
0 and 0(iv)
-2 and -2Q-4 What is the result of adding -8 and 3?
(i)
-5(ii)
-11(iii)
5(iv)
11Q-5 Which of the following statements is true?
(i)
The sum of two negative integers is positive.(ii)
The sum of a positive integer and a negative integer is always positive.(iii)
The sum of two integers can be zero.(iv)
The absolute value of a negative integer is always negative.Q-6 What is the predecessor of the integer 7?
(i)
6(ii)
8(iii)
0(iv)
-1Q-7 Which of the following integers is greater?
(i)
-3(ii)
-5(iii)
-1(iv)
-4Q-8 What is the result of subtracting -4 from 2?
(i)
-6(ii)
6(iii)
-2(iv)
2Q-9 If the temperature is -10°C and it rises by 5°C, what is the new temperature?
(i)
-5°C(ii)
5°C(iii)
-15°C(iv)
0°CQ-10 Which of the following integers is less than -2?
(i)
-1(ii)
0(iii)
-3(iv)
1