Question bank

Chapter-1   Rational Numbers
Q-1 Fill in the blanks:
(a) 2/3 is a ________ number.
(b) Every rational number is the quotient of _____ integers.
(c) -7/-12 is a _________ rational number.
Q-2 True or False:
(a) -7/8 is a negative rational number.
(b) -13/12 is a positive rational number.
(c) -5/3= -10/6

(d) Between any two distinct rational numbers, there are infinite rational numbers ____________.

(e) Between any two distinct integers, there is always an integer________.

(f) Between any two distinct rational numbers, there is always a rational number________.

Q-3 One word answer:
(a) LCM of 9 and 5.
(b) Which of the rational numbers 3/-4 and -5/6 is greater?
(c) The rational number which is neither positive nor negative.
Q-4

Express –3/5 as a rational number with denominator :

(i) 20

(ii) – 30

(iii) 35

(iv) – 40

Q-5

Solve:

1. Express –48/60 as a rational number with denominator 5.

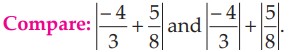
3. Find two rational numbers whose absolute value is 1/3 and 5/6 .

5. By what number should we multiply – 1/6 so that the product may be – 23/9 ?

6. The product of two rational numbers is 15. If one of the numbers is –10, find the other.

7. The cost of 2*1/3 metres of cloth is Rs.75*1/4 . Find the cost of cloth per metre.

8. The cost of 7*2/3 metres of rope is Rs.12*3/4 . Find its cost per metre.

9. The product of two rational numbers is —16/9 . If one of the numbers is – 4/5 , find the other.

10. Find a rational number between 1/4 and 1/3 .

11. Find two rational numbers between –2 and 2.

12. Find three rational numbers between – 7/2 and –2.

13. Find four rational number between 2/3 and 3/4 .

14. Find six rational numbers between –1 and 0.

15. Find ten rational numbers between –3/4 and 5/6 .

16. Insert ten rational numbers between –3/13 and 9/13.

17. Find any five rational numbers less than 2.

Q-6

Fill in the blanks:

(i) 7/–8 , expressed as a rational number with denominator 24 = .......................

(ii) –4/7 ................... 4/–11 (<,>,=).

(iii) The absolute value of –16/–19 = ...............

(iv) The rational number which is neither positive nor negative is …………….. .

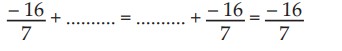
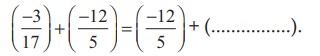
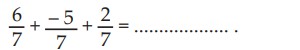
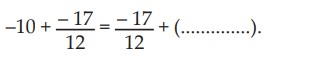
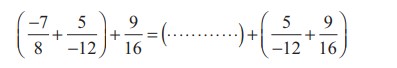
Q-7

Write four rational numbers equivalent to each of the following rational numbers:

(i) 2/5

(ii) 8/–11

(iii) –5/9

(iv) –12/13

Q-8

Write four rational numbers equivalent to each of the following rational numbers:

(i) –12/30

(ii) –14/49

(iii) 24/–64

(iv) –36/–63

Q-9

Which of the symbols <, = or > should replace the blank space?
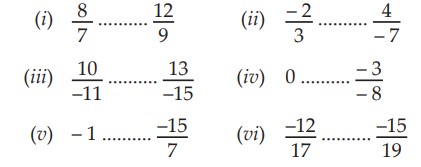
Q-10

Arrange the following rational numbers in ascending order:
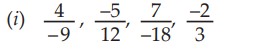
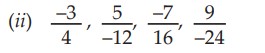
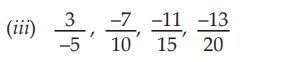
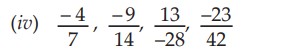
Q-11

Verify the following:
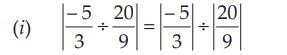
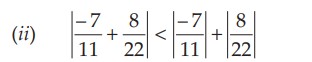
Q-12

Verify commutability of addition of rational numbers for each of the following pairs of rational numbers:

(i) – 11/5 and 4/7

(ii) 4/7 and 7/–12

(iii) – 3/5 and – 2/15

(iv) 2/–7 and 12/–35

Q-13

Find the additive inverse of each of the following:

(i) 1/3

(ii) 17/9

(iii) 15/–4

(iv) –16/–5

(v) –8/–7

Q-14

Verify the following:
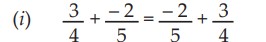
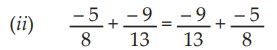
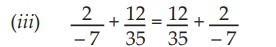
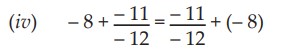
Q-15

Write True (T) or False (F) for the following:

(i) 0 is its own additive inverse______ .

(ii) The additive inverse of – 21/–30 is –21/30 ______ .

(iii) To subtract c/d from a/b , we add the additive inverse of a/b to c/d ______ .

(iv) While subtracting three or more rational numbers they can be grouped in any order _________.

(v) Subtracting is commutative for rational numbers _________.

Q-16

Write the negative (additive inverse) of each of the following :

(i) –2/5

(ii) 7/–9

(iii) –5/1

(iv) – 1

(v) –16/13

Q-17

Using the rearrangement property find the sum:

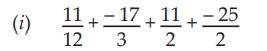
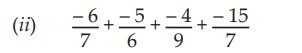
− 25/104

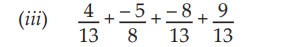
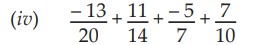
Q-18

Subtract:

(i) 4/9 from –1/6

(ii) 3/4 from 1/3

(iii) – 8/9 from – 3/5

(iv) 1/5 and 3/5

Q-19

Find each of the following products :

(i) 3/5 × –7/8

(ii) 25/–9 × 3/10

(iii) –2/5 and 10/–3

(iv) 16/–21 × 14/5

(v) – 2/24 × (–48)

(vi) – 2/3 × 6/7

(vii) – 7/6 × 24

(viii) – 6/11 × – 5/3

Q-20

Multiply:

(i) – 9/11 by 22/63

(ii) 18/35 by –1*1/8

(iii) 6/7 by 2/3

(iv) 5*1/7 by –2*1/3

Q-21

Simplify:

(i) –1/10 ÷ – 8/5

(ii) –12/7 ÷ (–18)

(iii) 4/9 ÷ – 3/12

(iv) –8 ÷ –17/16

Q-22

Verify each of the following:
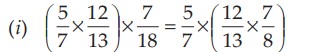
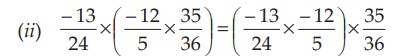
Q-23

Verify, whether each of the following are true or false:
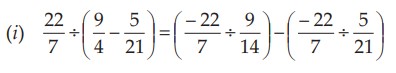
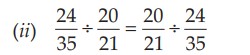
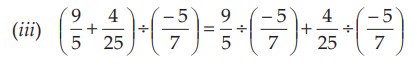
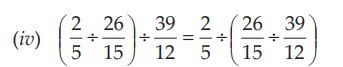
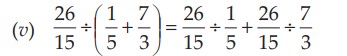
Q-24

Divide:

(i) 7/18 by –14/51

(ii) 10/33 by 2/–11

(iii) 5/9 by 15

(iv) –18 by (–36)/37

Q-25

Verify the following:
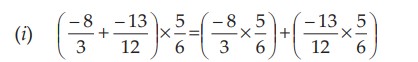
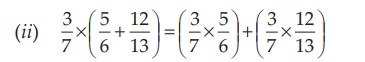
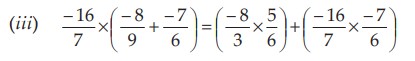
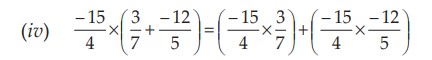
Q-26

Find the value of:
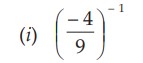
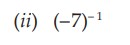
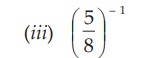
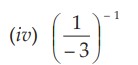
Q-27

Find (p + q) ÷ (p – q), if:

(i) p = 2/5 , q = 1/2

(ii) p = 1/4 , q = 3/2

(iii) p = 5/4 , q = – 1/3

(iv) p = 2/7 , q = 4/3

(v) p = 2/3 , q = 3/2

Q-28

Find a rational number between a and b, if:

(i) a = 1/8 , b = 7/12

(ii) a = –5/6 , b =– 2/5

(iii) a = 4/9 , b = 11/6

Multiple Choice Questions
Q-1 1, 2, 3, 4,..... are called _______ numbers.

(i)

natural

(ii)

composite

(iii)

none of these
Q-2 All fractions are ________ numbers.

(i)

rational

(ii)

irrational

(iii)

natural
Q-3 Every rational number is the quotient of _________ integers.

(i)

two

(ii)

three

(iii)

four
Q-4 A rational number is said to be negative if its numerator and denominator are of ______ signs.

(i)

same

(ii)

opposite

(iii)

none of these
Q-5 

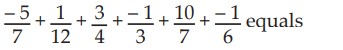
(i)

(a) 0

(ii)

(b) 1

(iii)

(c) 1*1/21

(iv)

(d) –1

Q-6 

Which of the following is not true?

(i)

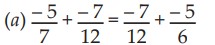
(ii)

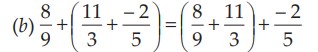
(iii)

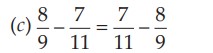
(iv)

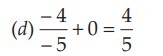
Q-7 

What number should be added to – 5/16 to get 7/24 ?

(i)

(a) – 29/48

(ii)

(b) 15/48

(iii)

(c) 29/48

(iv)

(d) 21/28

Chapter-2   Powers
Q-1 Fill in the blanks:
(a) In 3/4^2, 3/4 is the _________.
(b) In 3/4^2, 2 is the _________.
(c) 2^5 read as 2 raised to _________ 5.
Q-2 True or False:
(a) The result of 3^2 is 9.
(b) The result of 2^-3 is -8.
(c) The result of -1^5 is 1.
Q-3 One word answer:
(a) The reciprocal of 16/125.
(b) What is the result of (3/5^0)?
(c) What is the result of 3.74X10^2
Q-4

Express each of the following in exponential form :
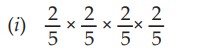
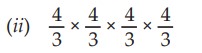
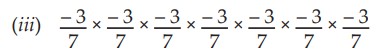
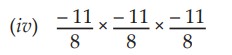
Q-5

Express each of the following as a rational number of the x/y , where x and y are integers and y ≠ 0:
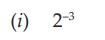
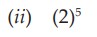
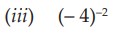
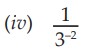
Q-6

Find the values of each of the following:
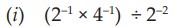
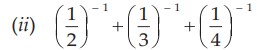
Q-7

Express each of the following rational numbers in power notation :
Q-8

Evaluate :
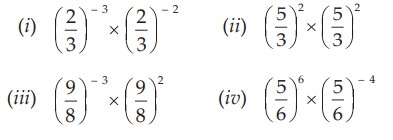
Q-9

Find the reciprocal of:

(i) 16/125

(ii) –27/64

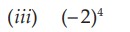
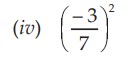
Q-10

Find the value of x for which
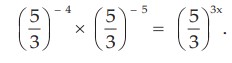
Q-11

Simplify:
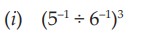
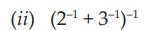
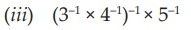
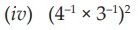
Q-12

Solve:
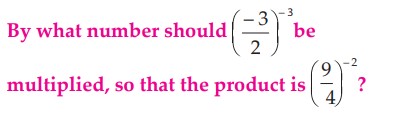
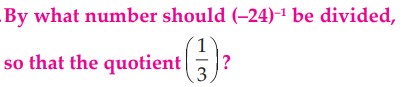
Q-13

Write each of the following numbers in standard form:

(i) 168000000

(ii) 3500000

(iii) 4630000000000

(iv) 273000

Q-14

Write the following numbers in the usual form:
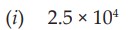
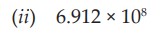
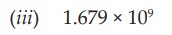
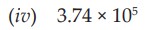
Q-15

Write each of the following numbers in the standard and usual form

(i) 0.00000000837

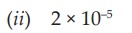
Q-16

Write the following numbers in the scientific notation:

(i) 0.0000000837

(ii) 0.003142

(iii) 0.00000542

(iv) 0.00002

Q-17

Fill in the blanks with <, > or = :
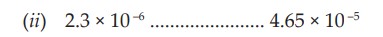
Multiple Choice Questions
Q-1 In 2^5, 2 is the _______.

(i)

base

(ii)

power

(iii)

exponent
Q-2 In 2^5, 5 is the _______.

(i)

power

(ii)

exponent

(iii)

both of these
Q-3 The reciprocal of 1/x^n is _______.

(i)

x^n

(ii)

x^-n

(iii)

x
Chapter-3   Squares and Square Roots
Q-1 Fill in the blanks:
(a) The square of 4 is _________.
(b) 9 is the square of _______.
(c) 4 is the square of ________.
Q-2 True or False:
(a) The result of 8^2 is 16
(b) The result of 10^2 is 100.
(c) 49 is the square of 9.
Q-3 One word answer:
(a) What is the square of 11?
(b) What is the square root of 144?
(c) What is the result of 6^2?
Q-4

Show that each of the following numbers is a perfect square. Also find the square numbers of the given numbers in each case:

(i) 1156

(ii) 2025

(iii) 4761

(iv) 14641

Q-5

Which of the following numbers are perfect square?

(i) 484

(ii) 625

(iii) 576

(iv) 941

(v) 2500

Q-6

Which of the following numbers are perfect squares ?

11, 12, 16, 32, 55, 36, 50, 64, 79, 81, 111, 121, 100, 105

Q-7

By what least number should the given numbers be multiplied to get a perfect square number? In each case, find the number whose square is the new number :

(i) 8820

(ii) 3675

(iii) 605

(iv) 2880

(v) 4056

(vi) 3468

(vii) 7776

Q-8

Solve:

1. Find the greatest number of two digits which is a perfect square.

2. Find the greatest number of three digits which is a perfect square.

3. Find the smallest number by which 1152 must be divided so that it becomes a perfect square. Also, find the number whose square is the resulting number.

4. Find the smallest number by which 4851 must be multiplied so that the product becomes a perfect squares.

5. Find the square root of 7.1824.

6. Express 64 as the sum of 8 odd numbers.

7. What number when multiplied by itself will become 83.7225 ?

8. Find the smallest number by which 5103 be divided to get a perfect square.

9. Find the least number which must be added to 8902 so that the sum becomes a perfect square.

10. The square root of 16 is 4. What is the number whose square root is 40?

12. The square root of 3 × 3 × 5 × 5 × 7 × 7 is?

13. What will be the square root of 14641?

14. The least number of four digits which is a perfect square is?

15. The square root of 16 is 4. What is the number whose square root is 40?

17. The square root of 3 × 3 × 5 × 5 × 7 × 7 is?

18. What will be the square root of 14641?

19. The least number of four digits which is a perfect square is?

20. The least number to be subtracted from 4568 so that the resulting number becomes a perfect square is ?

21. Write the square root of 0.8464.

22. The square root of 256/289 is?

23. Find the root of 3/7 correct to four places of decimal.

Q-9

Find the smallest number by which the given number must be divided so that the resulting number is a perfect square :

(i) 14283

(ii) 12150

(iii) 7688

Q-10

Simplify :
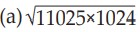
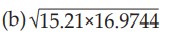
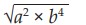
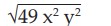
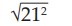
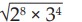
Q-11

Using the property, evaluate:
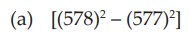
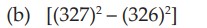
Q-12

Give reasons to show that none of the numbers given below is a perfect square :

(a) 782

(b) –160000

(c) 1530

(d) 2357

Q-13

State the number of digits in the square roots of the following squares :

(a) 848241

(b) 5041

(c) 15129

(d) 529

Q-14

Write down the units digits of the following squares :
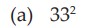
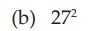
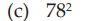
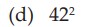
Q-15

Find Pythagorean triplets with the smallest number as :

(a) 10

(b) 12

Q-16

Find the square root by prime factorisation method :

(a) 7,056

(b) 2,91,600

(c) 7,744

(d) 6,084

Q-17

Find the square roots of the following numbers by division method :

(a) 54,289

(b) 6,889

(c) 8,281

(d) 3,969

Q-18

Evaluate :
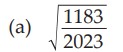
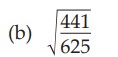
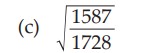
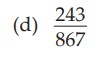
Q-19

Find square root of:

(a) 0.7225

(b) 156.25

(c) 1.96

(d) 225.6004

Q-20
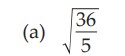
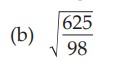
Q-21

Estimate the square root of the following to the nearest whole numbers :

(a) 259

(b) 75

(c) 460

Q-22

Find the square–roots of the following decimals :

(a) 13.69

(b) 1772.41

(c) 268.96

(d) 46.24

Multiple Choice Questions
Q-1 4 is the square of ________.

(i)

2

(ii)

3

(iii)

4
Q-2 25 is the square of _______.

(i)

3

(ii)

5

(iii)

7
Q-3 In general, square of a = a^2 = _____ for any number 'a'.

(i)

a*a

(ii)

a+a

(iii)

a/a
Q-4 Square numbers are also called ________.

(i)

perfect cubes

(ii)

perfect squares

(iii)

none of these
Q-5 

Which of these triplets form Pythagorean triplet?

(i)

(a) (4, 5, 6)

(ii)

(b) (6, 8, 10)

(iii)

(c) (10, 24, 26)

(iv)

(d) (7, 8, 10)

Q-6 

(i)

(a) 0.67

(ii)

(b) 8.2

(iii)

(c) 0.82

(iv)

(d) 0.935

Chapter-4   Cubes and Cube Roots
Q-1 Fill in the blanks:
(a) The cube of 1 is _______.
(b) The cube of 2 is ________.
(c) The cube of 7 is _________.
Q-2 True or False:
(a) The cube root of 216 is 6.
(b) 8 is not a perfect cube.
(c) The cube root of 64 is 4.
Q-3 One word answer:
(a) Is 648 a perfect cube?
(b) Is 27 a perfect cube?
(c) Is 1000 a perfect cube?
Q-4

Find the cube roots of the following numbers by prime factorisation method:

(i) 1738

(ii) 729

(iii) –74088

(iv) –64/125

(v) 2.744

(iv) 216 × 125

Q-5

Find the value of:
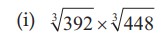
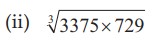
Q-6

Find the cube roots of the following:

(i) 729 × 216

(ii) 2744/35937

(iii) 4*473/2197

(iv) 0.085184

(v) 216/343

Q-7

Find the cubes of the following numbers :

(i) 8

(ii) 13

(iii) 103

(iv) –4/9

(v) 3/5

(vi) 27

(vii) – 2.4

(viii) 0.001

Q-8

Evaluate:
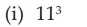
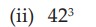
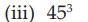
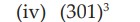
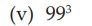
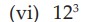
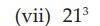
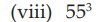
Q-9

Solve:

1. By what smallest number 29160 be divided so that the quotient becomes a perfect cube?

2. Find the volume of a cubical box whose side is 1.7 m.

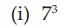
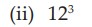
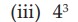
6. Find the cube roots of the number: 10,30,301 and 2197.

7. Is 256 a perfect cube? If so, find the cube root.

8. Volume of a cubical box is 46.656 m3 . Find the length of the side of the box.

9. What is the smallest number with which 7803 must be divided so that the quotient is a perfect cube? Also find the cube root of the quotient thus obtained.

10. What is the smallest number by which 784 be multiplied so that the product becomes perfect cube?

11. The surface area of a cube is 216 sq.cm. What is its volume?

12. How many cubes of side 2 cm can be packed in a cubical box with inner side equal to 4 cm?

Q-10

Find the odd numbers to be added to get the following cubes :
Q-11

Find the cube roots of the following :
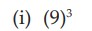
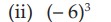
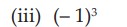
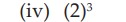
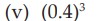
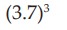
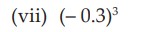
Q-12

Find the cube roots of the following:

(i) –729

(ii) –1728

(iii) –9261

(iv) –2197

Multiple Choice Questions
Q-1 What is the cube of 1?

(i)

1

(ii)

3

(iii)

5
Q-2 What is the cube root of 8?

(i)

1

(ii)

2

(iii)

8
Q-3 A natural number which can be expressed as a product of triplets of equal factors is known as a _______.

(i)

perfect square

(ii)

perfect cube

(iii)

none of these
Q-4 

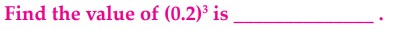
(i)

(i) 0.8

(ii)

(ii) 0.08

(iii)

(iii) 0.008

(iv)

(iv) 0.800

Q-5 

The cube of 70 is _______________ .

(i)

(i) 49,000

(ii)

(ii) 4,90,000

(iii)

(iii) 3,43,000

(iv)

(iv) 34,300

Q-6 

By which digit the cube of 27 ends?

(i)

(i) 1

(ii)

(ii) 2

(iii)

(iii) 4

(iv)

(iv) 3

Q-7 

Cube of (2 – 1/3 ) is _______________.

(i)

(i) 8 – 1/27

(ii)

(ii) 125/27

(iii)

(iii) 25/9

(iv)

(iv) 343/47

Q-8 

The smallest number by which 3645 be multiplied so that the product becomes a perfect cube is :

(i)

(i) 5

(ii)

(ii) 25

(iii)

(iii) 15

(iv)

(iv) 35

Chapter-5   Algebraic Expressions
Q-1 Fill in the blanks:
(a) A combination of constants and variables connected by fundamental operations that is, +, –, ×, and ÷ signs, is known as an __________ expression.
(b) 5a, 3x + 7, and 5x2 + 3y – 8 are _________ expressions.
(c) x, y, and a are called _________ numbers, or variables, as they can take various numerical values.
Q-2 True or False:
(a) 5a has one term.
(b) 3x+7 has two terms.
(c) 5x2 + 3y – 8 has four terms.
Q-3 One word answer:
(a) How many terms are there in binomial expression?
(b) How many terms are there in trinomial expression?
(c) How many terms are there in monomial expression?
Q-4

Which of the following expressions are polynomials?
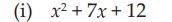
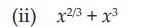
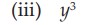
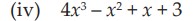
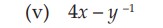
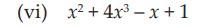
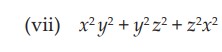
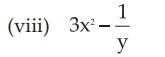
Q-5

Classify the following polynomials into monomials, binomials and trinomials :
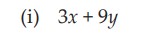
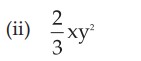
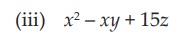
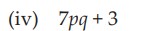
Q-6

State the degrees of the following polynomials:
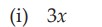
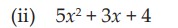
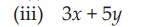
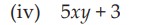
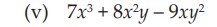
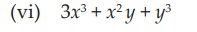
Q-7

Add:
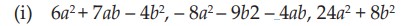
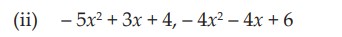
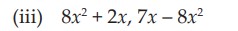
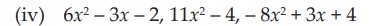
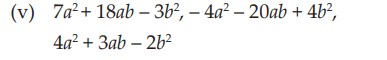
Q-8

Subtract :
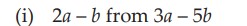
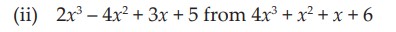
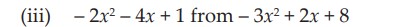
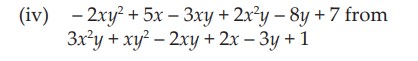
Q-9

Solve:
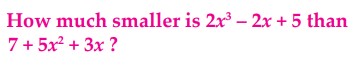
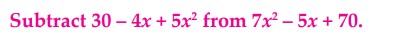
Q-10

Add the following polynomials :
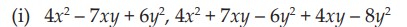
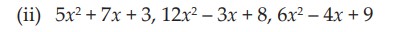
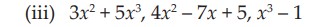
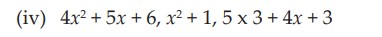
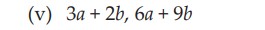
Multiple Choice Questions
Q-1 A monomial expression has ________ term.

(i)

one

(ii)

two

(iii)

three
Q-2 A binomial expression has ________ terms.

(i)

two

(ii)

three

(iii)

four
Q-3 A trinomial expression has _______ terms.

(i)

one

(ii)

two

(iii)

three
Q-4 An algebraic expression having one or more terms is called a ________.

(i)

binomial

(ii)

trinomial

(iii)

polynomial
Chapter-6   Factorisation of Algebraic Expressions
Q-1 Fill in the blanks:
(a) ___________ is the reverse process of multiplication.
(b) Factors of a __________ consist of every literal, their product and number that will divide it exactly.
(c) 1 is a factor of every algebraic term, so 1 is called a ________ factor.
Q-2 True or False:
(a) The HCF of 6 and 24 is 6.
(b) The process of finding factors of a given expression is called factorisation.
(c) Factorisation can be done by taking out a common factor and by making groups.
Q-3 One word answer:
(a) a^2+B^2+2ab is the formula of ?
(b) a^2+b^2-2ab is the formula of?
(c) Which is the coefficient of ax+9?
Multiple Choice Questions
Q-1 Factorisation is the reverse process of ______________.

(i)

multiplication

(ii)

division

(iii)

addition
Q-2 The process of writing a given algebraic expression as the product of two or more factors is called _______.

(i)

multiplication

(ii)

factorisation

(iii)

none of these
Q-3 Finding factors is ________ of finding products.

(i)

same

(ii)

opposite

(iii)

both of these
Chapter-7   Linear Equations in one Variable
Q-1 Fill in the blanks:
(a) An __________ is a statement of equality of two algebraic expressions involving one or more variables.
(b) An equation which contains one or more variables with maximum power as _______ is called a linear equation.
(c) If a linear equation has only _______ variable it is called a linear equation in one variable.
Q-2 True or False:
(a) The value of the variable which satisfies the equation is called a solution or a root of the equation.
(b) The LCM of 4,3,3 is 12.
(c) The degree of the polynomial involved in a linear equation is two.
Q-3 One word answer:
(a) What is the solution of the equation 9x – 1 = 8?
(b) If 2x+9=15, then what is the value of x?
(c) In 2x + 4 = x – 5, what is the value of x?
Multiple Choice Questions
Q-1 An _____________ is a statement of equality of two algebraic expressions involving one or more variables.

(i)

algebra

(ii)

equations

(iii)

none of these
Q-2 An equation which contains one or more variables with maximum power as 'one' is called a _______ equation.

(i)

binomial

(ii)

trinomial

(iii)

linear
Q-3 The value of a variable that satisfies the equation is called a ________.

(i)

answer

(ii)

quotation

(iii)

solution
Chapter-8   Representing Solid Shapes on Paper
Q-1 Fill in the blanks:
(a) ________ dimensional shapes are called in brief 2-D shapes.
(b) When a solid object has length, breadth and height as dimensions, then it is called _________ dimensions object.
(c) There are ______ views of a solid object.
Q-2 True or False:
(a) There are mainly four types of solids.
(b) Top view is a view in solid object.
(c) A cuboid is also called a triangular prism.
Q-3 One word answer:
(a) What is also known as tetrahedron?
(b) Which pyramid's base is a rectangle?
(c) How many vertices are there in rectangular pyramid?
Multiple Choice Questions
Q-1 Every solid objects has ______ different views.

(i)

two

(ii)

three

(iii)

four
Q-2 There are mainly _________ types of solids.

(i)

two

(ii)

three

(iii)

four
Q-3 Cone is a special type of ________.

(i)

rectangle

(ii)

square

(iii)

pyramid
Q-4 ________ is a solid whose every point equidistant from a fixed point.

(i)

Pyramid

(ii)

Sphere

(iii)

Prism
Chapter-9   Compound Interest
Q-1 Fill in the blanks:
(a) When time is given in months, we convert it in to year by dividing it by ___________.
(b) The duration for which the interest is calculated is called the __________ period.
(c) The conversion period is ______ year.
Q-2 True or False:
(a) When the interest is added to the principal at the end of each year, we say that the interest is compounded annually.
(b) Compound Interest is calculated on accumpulated interest and principal interest.
(c) Simple interest original amount deposited or borrowed.
Q-3 One word answer:
(a) It is calculated on principal at fixed rate of interest and time.
(b) It is calculated on accumpulated interest and principal interest.
(c) Sum of principal and simple interest.
Multiple Choice Questions
Q-1 The money borrowed or lent is called _________.

(i)

principal

(ii)

interest

(iii)

rate
Q-2 The person from whom the money is borrowed is called the __________.

(i)

lender

(ii)

borrower

(iii)

none of these
Q-3 The person who borrows the money is called the __________.

(i)

lender

(ii)

borrower

(iii)

none of these
Q-4 When time is given in days, we convert it in to year by dividing it by _______.

(i)

24

(ii)

365

(iii)

366
Chapter-10   Percentage and Its Applications
Q-1 Fill in the blanks:
(a) Percentages, Fractions and Decimals are __________ to each other.
(b) ______ can be written as 20/100.
(c) To convert a fraction into a percent, we multiply the fraction by _______.
Q-2 True or False:
(a) 35% can be written as 35/100 or 7/20.
(b) 25/100 is the fraction of 25%.
(c) 15% of 100 is 12.
Q-3 One word answer:
(a) What is the 20% of 200?
(b) What is the 13% of 100?
(c) What is the 10% of 2000?
Multiple Choice Questions
Q-1 The term percent means per __________ or for every hundred.

(i)

hundred

(ii)

thousand

(iii)

million
Q-2 ___________ is always calculated on the marked price of the item.

(i)

Discount

(ii)

Interest

(iii)

none of these
Q-3 Sales tax is paid in a state to the respective ________ government.

(i)

central

(ii)

union

(iii)

state
Q-4 The prices of the articles include the tax, called _______.

(i)

sales tax

(ii)

VAT

(iii)

none of these
Chapter-11   Practical Geometry (Construction)
Q-1 Fill in the blanks:
(a) Which of the following lies between 0 and –1 on a number line?
(b) How many measurements are necessary to construct a quadrilateral uniquely?
(c) How many diagonals are there in a hexagon?
Q-2 True or False:
(a) The sum of all interior angles of a regular hexagon is 7200.
(b) A pentagon has four diagonals.
(c) The sum of interior angles of a quadrilateral is 360°.
Multiple Choice Questions
Q-1 Quadrilaterals are constructed by splitting up the figure into two __________.

(i)

rectangles

(ii)

triangles

(iii)

circles
Q-2 A quadrilateral has __________ parts in all.

(i)

six

(ii)

eight

(iii)

ten
Q-3 A quadrilateral has ________ angles.

(i)

three

(ii)

four

(iii)

five
Chapter-12   Mensuration
Q-1 Fill in the blanks:
(a) Octagon has _________ sides.
(b) A regular polygon has all its sides and angles _________.
(c) A triangle has _________ sides.
Q-2 True or False:
(a) A rectangle has four sides.
(b) A square has four sides.
(c) A hexagon has six sides.
Q-3 One word answer:
(a) What fits directly to a circle?
(b) In how many equilateral triangles can regular hexagon be divided?
(c) Write the formula of surface area of a cube.
Multiple Choice Questions
Q-1 A plane figure having three or more sides is called a ___________.

(i)

rectangle

(ii)

polygon

(iii)

square
Q-2 A triangle has _______ sides.

(i)

two

(ii)

three

(iii)

four
Q-3 A regular polygon has all its sides and angles ________.

(i)

equal

(ii)

unequal

(iii)

none of these
Chapter-13   Direct and Inverse Variations
Q-1 Fill in the blanks:
(a) 2 pencils cost Rs. 8 then 4 pencils will cost Rs. __________.
(b) There are ______ types of variations.
(c) x/y is always a _______ number.
Q-2 True or False:
(a) x/y or k is called the constant of variation.
(b) There are 1000X1000 cubic metres in 1 litre.
(c) Speed is the ratio of time and velocity.
Q-3 One word answer:
(a) If 40 metres of a cloth costs Rs. 1940. How many metres can be bought for Rs. 727.5?
(b) A shopkeeper has just enough money to buy 52 cycles worth Rs. 525 each. If each cycle were to cost Rs. 21 more than the amount he has, how many cycles would he be able to buy?
Multiple Choice Questions
Q-1 There are two types of variations : _________________.

(i)

Direct and Indirect

(ii)

Direct and Inverse

(iii)

both of these
Q-2 x/y is always a ____________ number.

(i)

positive

(ii)

negative

(iii)

constant
Q-3 x/y or k is called the _________ of variation.

(i)

constant

(ii)

positive

(iii)

negative
Q-4 how many types of variation are there.

(i)

2

(ii)

3

(iii)

4
Chapter-14   Playing with Numbers
Q-1 Fill in the blanks:
(a) A two-digit number 10a + b is divisible by _______ if ‘b’ is either 0 or 5.
(b) A number is divisible by 2 if its last digit is ________.
(c) A three-digit number 100a + b + c is divisible by ___________, if ‘c’ is divisible by 5.
Q-2 True or False:
(a) A number is divisible by 3, if the sum of its digits is not divisible by 3.
(b) A number is divisible by 9 if the sum of its digits is not divisible by 9.
(c) A number divisible by 9 is also divisible by 3.
Q-3 One word answer:
(a) Can the first digit of a number be zero?
(b) Is 20 divisible by 10?
(c) Is 53 divisible by 2?
Multiple Choice Questions
Q-1 A _____________ is said to be in a generalised form.

(i)

number

(ii)

digit

(iii)

units
Q-2 A number divisible by 9 is also divisible by ________.

(i)

4

(ii)

3

(iii)

5
Q-3 Each letter (alphabets) in a ___________ must stand for just one digit.

(i)

number

(ii)

puzzle

(iii)

digit
Chapter-15   Data Handling
Q-1 Fill in the blanks:
(a) The word data means _________.
(b) The collection of all the observation is called __________.
(c) __________ is the science which deals with the collection, presentation, analysis and interpretation of numerical data.
Q-2 True or False:
(a) Frequency distribution is of two types.
(b) Frequency is the number of times a particular event occurs.
(c) Data can be arranged and presented by grouped frequency distribution.
Q-3 One word answer:
(a) In what, there is no gap?
(b) What shows the relationship between a whole and its parts?
Multiple Choice Questions
Q-1 We need information in the form of __________ figures.

(i)

numerical

(ii)

alphabetical order

(iii)

raw order
Q-2 The collection of all the observation is called __________.

(i)

rat data

(ii)

statistical data

(iii)

data
Q-3 Each numericals fact of this types is known as an _____________.

(i)

data

(ii)

observation

(iii)

numerical observations
Q-4 It is the science which deals with the collection, presentation, analysis and interpretation of ____________.

(i)

numerical data

(ii)

statistical data

(iii)

none of these
Chapter-16   Probability
Q-1 Fill in the blanks:
(a) _________ means the chance of happening of certain things in uncertain circumstances.
(b) The situation of __________ can be estimated numerically by means of probability.
(c) An experiment is called a ___________ experiment if all its possible outcomes are known but the result cannot be predicted in advance.
Q-2 True or False:
(a) An event is one or more outcomes of an experiment.
(b) Getting number 3 when a dice is rolled is not an event.
(c) An outcome is the result of a single trial or event of an experiment.
Q-3 One word answer:
(a) What is the collection of all possible outcomes of a random experiment called?
(b) Sample space is denoted by ________.
(c) How many possible outcomes are there, when a dice is rolled?
Multiple Choice Questions
Q-1 Probability means the chance of happening of certain things in uncertain ______________.

(i)

circumstances

(ii)

uncertainty

(iii)

situation
Q-2 An event is one or more outcomes of an _____________.

(i)

collection

(ii)

experiment

(iii)

none of these
Q-3 An experiment is called a ____________ experiment.

(i)

Event

(ii)

Probability

(iii)

random
Q-4 The collection of all possible outcomes of a random experiment is called its _____________.

(i)

sample space

(ii)

random space

(iii)

event space
Chapter-17   Introduction to Graphs
Q-1 Fill in the blanks:
(a) The distance OM of the point P from the y-axis is called x-coordinate or _______.
(b) The distance PM of the point P from the x-axis is called the y-coordinate or _________.
(c) The coordinate axes divide the plane of the graph paper into four regions, called _____________.
Q-2 True or False:
(a) The coordinates of the origin obviously are (o, o).
(b) Point (-2,4) lies in 2nd quadrant.
(c) Point (3,5) lies in 1st quadrant.
Multiple Choice Questions
Q-1 Pictorial and ___________ representations are easier to understand.

(i)

graphical

(ii)

Coordinate Axes

(iii)

origin
Q-2 The plane of the paper containing both the coordinate axes is called the _____________.

(i)

Coordinate Axes

(ii)

Cartesian plane

(iii)

ordinate plane
Q-3 For fixing a point on the graph sheet we take two mutually perpendicular lines called _________.

(i)

origin

(ii)

axis

(iii)

axes